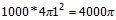XAT 2012 : Quant Question 66 : Mensuration Surface Area

Question

A spherical metal of radius 10 cm is molten and made into 1000 smaller spheres of equal sizes. In this process the surface area of the metal is increased by:

A.    1000 times
B.    100 times
C.    10 times
D.    No Change
E.    None of the above

Correct Answer : 10 times. Choice C

Explanatory Answer
Let the radius of the small spheres be ‘r’ cm.
The amount of metal remains the same in both cases.

Hence, volume of the spherical metal of radius 10 cm should be equal to the volume of the 1000 smaller spheres of radius ‘r’ cm put together.

Therefore, r = 1 cm.
Surface area of a spherical metal of radius 10 cm is

 


Surface area of 1000 smaller spheres put together is

Thus the surface area increased by 10 times.

XAT 2012 Quant Q65 : Siimple Interest

This question appeared in the XAT 2012 quant section. Question 65.

Question

A man borrows Rs. 6000 at 5% interest, on reducing balance, at the start of the year. If he repays Rs. 1200 at the end of each year, find the amount of loan outstanding, in Rupees, at the beginning of the third year.

A. 3162.75
B. 4125.00
C. 4155.00
D. 5100.00
E. 5355.00

Correct Answer : Choice C. Rs.4155.00

Explanatory Answer
Interest payable @ the end of the first year @ 5% interest on Rs 6000 =

Amount payable @ the end of the first year = Rs 6000 + Rs 300 = Rs 6300
Amount paid @ the end of the first year = Rs 1200
Therefore, Principal for the second year = Rs 6300 – Rs 1200 = Rs 5100

Interest payable @ the end of the second year @ 5% interest on Rs 5100 =

Amount payable @ the end of the second year = Rs 5100 + Rs 255 = Rs 5355
Amount paid @ the end of the second year = Rs 1200
Therefore, Principal for the third year = Rs 5355 – Rs 1200 = Rs 4155

Thus, the amount of loan outstanding at the beginning of the third year = Rs 4155.

XAT 2012 Q64 - Mensuration 1 Mark

Question

Ram a farmer, managed to grow shaped-watermelons inside glass cases of different shapes. The shapes he used were: a perfect cube, hemi-spherical, cuboid, cylindrical along with the normal spherical shaped watermelons. Thickness of the skin was same for all the shapes. Each of the glass cases was so designed that the total volume and the weight of the all the water-melons would be equal irrespective of the shape.

A customer wants to buy water-melon for making juice, for which the skin of the water-melon has to be peeled off, and therefore is a waste. Which shape should the customer buy?

A.Cube
B.Hemi-sphere
C.Cuboid
D.Cylinder
E.Normal spherical
Correct Answer : Choice E - Normal Spherical

Explanatory Answer

One of those questions that is either very easy or very difficult depending on whether you know the concept that is tested.

For a given surface area, the volume contained increases with increasing symmetry of the object. For instance, if we are to make water melons of different shapes of the same surface area, the volume will be maximum when it is made into a sphere.

The corollary is that for a given volume, the surface area will be minimum when the object is a sphere. So, the customer should opt for spherical shaped water melons if she has to minimize wastage.

For 2-dimensional object, for a given perimeter, the area increases with increasing symmetry.
Among different triangles of a given perimeter, an equilateral triangle has the largest area.

The area increases with increasing number of sides - i.e., for a given perimeter the area of a square will larger than that of an equilateral triangle; the area of a regular pentagon of a given perimeter will be larger than that of a square and so on.

Among different regular polygons of a given perimeter / circumference a circle has the largest area.

XAT 2013 Registration Notification Announced

The advertisement notifying XAT 2013 dates and online registration window was released in leading dailies on August 8, 2012.

 

Key information of use to XAT test takers.

 

XAT 2013 : Sunday, Jan 6, 2013.

Online registrations for the test start: August 20, 2012

Online registrations for the test end: November 30, 2012

 

XAT test fees : Rs.950 for general students and Rs.650 for SC / ST students.

Website for online registration : http://www.xatonline.net.in

 

Usually about 3 months of dedicated preparation for the XAT will result in getting a good percentile and an admit into one of the leading programs that admit students through the XAT process.

 

Over 100 management institutes / business schools in India admit students through the Xavier Aptitude Test (XAT). Leading among them include XLRI, Jamshedpur, XIM Bhubaneswar, XIME Bangalore, XISS, LIBA Chennai, and St. Joseph's Bangalore.

XAT 2012 Qn 63 - Percents, Ratio

This is a very easy 1 mark question that appeared in XAT 2012. The question is from the topic percents.

Question 63 of XAT 2012

Tina, Mina, Gina, Lina and Bina are 5 sisters, aged in that order, with Tina being the eldest. Each of them had to carry a bucket of water from a well to their house. Their buckets’ capacities were proportional to their ages. While returning, equal amount of water got splashed out of their buckets. Who lost maximum amount of water as a percentage of the bucket capacity?
A.    Tina
B.    Mina
C.    Gina
D.    Lina
E.    Bina

Correct Answer - Bina. Choice E.

Explanatory Answer

Tina is the oldest and Bina is the youngest.
So, Bina’s bucket would have been the smallest.
   
Each sister lost equal amount of water.

As a proportion of the capacity of their buckets Bina would have lost the most.

Alternatively, you could solve this question by assuming some numbers for the capacity of the buckets that are in descending order from Tina to Bina and a value for the quantity that was lost and compute the percentages and verify.

You could access more XAT Practice questions on Percentage by clicking here..

XAT 2012 Q59 : Mean, Median, Mode

This is a 1 - Mark question that appeared in XAT 2012 from Statistics. This is an interesting question that tests your basic understanding of how Mean, Median and Mode can be interpreted.

Question

Ramesh analysed the monthly salary figures of five vice presidents of his company. All the salary figures are in integer lakhs. The mean and the median salary figures are Rs. 5 lakhs, and the only mode is Rs. 8 lakhs. Which of the options below is the sum (in Rs. lakhs) of the highest and the lowest salaries?
A.    9
B.    10
C.    11
D.    12
E.    None of the above

Correct Answer – Choice A. Rs. 9 lakhs

Explanatory Answer

The mean salary of the five vice presidents is Rs.5 lakhs.
So, the sum of their salaries = 5 * 5 = 25 lakhs.

Let their salaries in ascending order be a, b, c, d and e.
So, a + b + c + d + e = 25.
The median salary is Rs.5 lakhs. So, C’s salary is Rs.5 lakhs.

The only  mode is Rs.8 lakhs.
So,  Rs.8 lakhs salary is drawn by the maximum number of VPs.
C’s salary is Rs.5 lakhs. So, d and e have to draw Rs. 8 lakhs each.

Therefore, a + b + 5 + 8 + 8 = 21
or a + b = 4

Their salaries are in integer lakhs.
Therefore, a can draw Rs.1 lakh and b can draw Rs.3 lakhs or a and b can both draw Rs. 2 lakhs each.

However, there is only one mode. So, a and b cannot draw Rs.2 lakhs each.
So, a draws Rs.1 lakh (the least salary) and e draws Rs.8 lakhs (the highest salary).

So, a + e = Rs.9 lakhs

Click for additional Mean, Median and Mode questions

XAT 2012 Quant - Number Properties: LCM

Please watch this blog for periodic updates on new posts of previous year XAT questions with answers and explanation.

You could register you mobile number at http://labs.google.co.in/smschannels/channel/XATQuestions to receive questions by SMS. This service is available to any Indian mobile number that is not registered with National Do Not Call registry and is offered free of cost.

XAT 2012 Question 58  : LCM Word Problem

 Three Vice Presidents (VP) regularly visit the plant on different days. Due to labor unrest, VP (HR) regularly visits the plant after a gap of 2 days. VP (Operations) regularly visits the plant after a gap of 3 days. VP (sales) regularly visits the plant after a gap of 5 days. The VPs do not deviate from their individual schedules. CEO of the company meets the VPs when all the three VPs come to the plant together. CEO is on leave from January 5th to January  28th, 2012. Last time CEO met the VPs on January 3, 2012. When is the next time CEO will meet all the VPs?
A.    February 6, 2012
B.    February 7, 2012
C.    February 8, 2012
D.    February 9, 2012
E.    None of the above

Correct Answer February 8, 2012.

Explanatory Answer

VP (HR) visits the plant every 3rd day (the question states the VP visits after a gap of 2 days). 

The most important point in this question is this point. As the VP visits the plant after a gap of 2 days - he visits the plant every third day - not every second day.


If VP (HR) visited the plant on day 0, he will visit again on day 3, day 6 and so on.
i.e., on days that are multiples of 3.

VP (Operations) visits the plant every 4th day (as he visits after a gap of 3 days).
If VP (Operations) visited the plant on day 0, he will visit again on day 4, day 8 and so on.
i.e., on days that are multiples of 4.

VP (Sales) visits the plant every 6th day (as the VP Sales visits after a gap of 5 days).
If VP (Sales) visited the plant on day 0, he will visit again on day 6, day 12 and so on.
i.e., on days that are multiples of 6.

So, all 3 VPs will visit the plant together again on a day that is a multiple of 3, 4 and 6 or on a day that is a common multiple of 3, 4 and 6.

The first such common multiple is the LCM of 3, 4 and 6 = 12.

The last date on which all three VPs met the CEO was on Jan 3, 2012.
The next dates on which their visits will coincide will be after 12 days and multiples therefore. 
i.e., on Jan 15, 2012, Jan 27, 2012, Feb 8, 2012.

The CEO is on leave from Jan 5, 2012 to Jan 28, 2012. 
So, the CEO will meet the VPs on February 8, 2012.